Carbon Cycle Processes
(relevant for timescales of a few hundred years)
Processes of Flow in the Terrestrial Realm
- Photosynthesis
- Plant Respiration
- Litter Fall and Below-Ground Addition
- Soil Respiration
- Runoff
Processes of Flow in the Oceanic Realm
- Ocean-Atmosphere Exchange
- Carbonate Chemistry of Seawater
- Summary of Carbonate Chemistry
- Ocean-Atmosphere Exchange Revisited
- Advection -- Surface Currents
- Marine Biota Exchange -- The Biologic Pump
- Downwelling
- Upwelling
- Sedimentation
- Volcanism and Metamorphism
Processes of Carbon Flow in the Human Realm
- Fossil Fuel Burning
- Land-Use Changes -- Forest Burning and Soil Disruption
Other Sections of the Carbon Cycle Chapter
- I. Introduction to the Carbon Cycle
Before we embark on our adventure of modeling the global carbon cycle, it is important to point out that the present-day carbon cycle is not in a steady state. This means that if, as is typical, we want to begin by creating a steady-state model that will serve as our experimental control, we should not force our model to mimic the present day situation. Instead, we will use the carbon cycle as it is believed to have existed (somewhat closer to a steady state) in the time just before the onset of the industrial revolution, which marks the time when human alterations of the carbon cycle began in earnest. Adopting this pre-industrial case as our steady state has another advantage in that we know the history of CO2 emissions from human activities pretty well and we know the present state of the carbon cycle pretty well and we even know the rate of change of various parts of the carbon cycle. Knowing these things about the carbon cycle, we can perform a very good test on our model of the carbon cycle. If we add to our steady state model the CO2 emission history for the last 100 years, we should end up with a carbon cycle in a state very similar to today's . This will be provide us with a very nice way of assessing the significance of our modeling results.
To begin, we will make a survey of the various processes involved in the carbon cycle, dividing these processes into those that occur on land (the terrestrial realm), those that occur primarily in the oceans, and those related to human activities.
Processes of Carbon Flow in the Terrestrial Realm
Since it's invention over 3 billion years ago, photosynthesis has been one of the most important processes on Earth, helping to make our planet different from all the other planets. The basic idea is that plants capture light energy and use it to split water molecules and then combine the products with carbon dioxide to make carbohydrates, which are used for fuel and construction of the plant; oxygen is a by-product of this reaction, which is summarized as:
This process takes places in the chloroplasts located in the interiors of leaves. Here, chlorophyll absorbs solar energy in the red and blue parts of the spectrum. This energy is then used to split a water molecule into hydrogen and oxygen; in the process, the plants gain chemical energy that is used in a companion process that converts carbon dioxide into carbohydrates represented by CH2O in the above equation.
The CO2 used in this process gets into the interior of the leaf through small openings about 10 microns in diameter called stomata, which the plant can control like a valve, opening and closing to adjust the rate of transfer. The rate of CO2 transfer is also a product of how much CO2 is inside the leaf. For instance, if the interior of a leaf was filled with lots of CO2, then even if the stomata were wide open, little new CO2 could enter the leaf -- it would be limited by how quickly the plant was consuming CO2 in photosynthesis. Plants that are actively photosynthesizing generally have their stomata wide open, but there is sometimes a problem with this because with the stomata open, water from inside the plant escapes out into the air. As a consequence, when water is scarce, plants tend to close their stomata and thus they cannot photosynthesize at the maximum rate. Photosynthesis is also limited by the availability of other nutrients, especially nitrogen, which tends to be a limiting nutrient in many terrestrial ecosystems.
However, as a general rule, if plants do have sufficient water, they will increase their rate of photosynthesis if there is more CO2 in the air surrounding them -- an effect known as CO2-fertilization. An interesting thing happens when the rate of photosynthesis increases; the leaf interiors are filled with more CO2, so the stomata close down to limit the intake of more CO2, and in doing this, the plant also limits the amount of water that can escape. What this amounts to is an increased efficiency of water use by the plants -- they grow more while using less water. But, there is an upper limit to this effect; at high levels of CO2, plants do not respond to additional increases in atmospheric CO2. Likewise, there is a lower limit to photosynthesis imposed by CO2 levels, but we are not likely to approach such low levels in the next billion years or so (we will eventually have this problem since atmospheric CO2 is slowly being drawn down through the precipitation of limestones).
Temperature is another important consideration in many life processes, and photosynthesis is no exception. As a general rule, the rates of most metabolic processes increase with temperature, but there is usually an upper limit where the high temperatures begin to destroy important enzymes, or otherwise inhibit life functions. For the majority of plants, this upper limit is not likely to come into play given the kinds of temperature changes we might expect in the space of a couple hundred years, so we can safely ignore it here (if our model does lead to temperature changes of greater than 10-20°C in a hundred years, we would presume that there is some problem with the model as this is unrealistic behavior). Another important reason to take temperature into account in our photosynthesis flow is that it turns out that in most environments, an increase in temperature correlates with an increase in precipitation, and since many regions where plants grow are somewhat limited by water, especially towards the end of the growing season, increased precipitation leads to a greater yearly rate of photosynthetic uptake of atmospheric carbon.
How will we keep track of the changing temperature? Recall from our modeling of the solar energy system, in an earlier chapter, that increase atmospheric CO2 leads to an increase in the greenhouse effect and thus a warmer planet. One option is that we could attach our model of the carbon cycle to one of our models for the solar energy system. Another, simpler option is to develop a simple relationship based on the known history of atmospheric CO2 and global temperature. This has been done by a variety of people, and the result is an equation that looks like this:
Where DT is the temperature difference, in °C, between the start and any time, t, during the model simulation; 280 ppm (parts per million) is the beginning concentration of CO2 in the atmosphere, representing the pre-industrial world. In this way, we monitor the temperature relative to the starting temperature (which is 15°C). Note that using this equation, doubling atmospheric CO2 would result in a 2.8°C rise -- in the range of estimates provided by computer models.
Now, we must consider the problem of how to describe photosynthesis, globally, in the form of an equation that we can use in a model. We do get some help here from studies on a variety of different plants grown in greenhouses with varying levels of CO2 and varying temperatures. We should realize, of course, that there are always uncertainties that arise when transferring results from the laboratory to the real world, and there are even more problems when you try to extrapolate to the scale of the whole world. The truth of the matter is that we don't know enough about the operation of our whole planet to solve this problem with confidence -- the study of the global carbon cycle is in its early stages. And yet if we don't make some attempt to describe this process in the form of a global model, our understanding of the dynamics of the global carbon cycle will languish in the early stages. So we forge ahead.
The accumulated results indicate that our function for photosynthesis should satisfy the following characteristics:
1) the global flow should be 100 Gt C/yr (one gigaton of carbon per year; 1Gt = 1015g)2) the rate should increase at higher atmospheric CO2 level, but there should be a saturation point, where additional increases in atmospheric CO2 yield no further increase in the rate of photosynthesis
3) the rate should increase with increasing temperature.
There are several ways to construct an equation that incorporates this information, but here, we use the following:
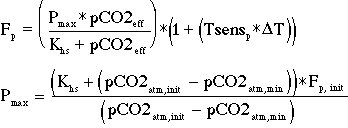
Fp is the global rate of photosynthetic uptake of CO2 in GtC/yr.
Pmax is a parameter with units of GtC/yr that is used to force the equation for Fp to give the proper value corresponding to the starting conditions of the model.
Khs = 62.5 ppm -- the half-saturation value -- the atmospheric CO2 concentration at which the rate of photosynthesis, Fp, is half of the ultimate saturation value, given that particular temperature
pCO2atm,init = 280 ppm -- the pre-industrial atmospheric CO2 concentration
pCO2atm,min = 30 ppm -- value below which no photosynthesis can occur
pCO2eff = pCO2atm - pCO2atm,min-- the effective atmospheric CO2 concentration
Fp,init = 100 GtC/yr -- the initial value for global photosynthesis
Tsensp = 0.04 -- establishes the increase of Fp per degree of warming
DT = T - Tinit -- temp. difference relative to initial temp., Tinit is set to 0
Figure 7.04 shows what the first part of the equation for Fp looks like; here you can see that our equation for photosynthesis is similar to an exponential function, though to be more precise, this is called a rectangular hyperbola.
We could just as easily devise a way of using graphical converters in
STELLA to do the same job, but this equation will produce a smoother
function and provides us with greater flexibility in modifying it
during sensitivity analyses. The Khs
parameter, the so-called half-saturation value, is the atmospheric
CO2 concentration that corresponds to a rate of
photosynthesis (Fp) that is half the ultimate saturation
value (see Figure 7.04). Khs
is a key parameter in defining the shape of the curve, which is
always constrained to give a photosynthesis rate of 100 Gt C/yr when
the atmospheric CO2 concentration is 280 ppm -- the
pre-industrial value. This means that in our standard case
(Khs
= 62.5 ppm) when the CO2 -fertilization effect
("greening") reaches its limit, the rate of photosynthesis will be
125 Gt C/yr -- a 25% increase over the starting value.
The temperature sensitivity part of the equation is simply a linear function, meaning that if you plot the temperature sensitivity versus the temperature, you get a straight line. The formulation here gives an increase in photosynthesis by a factor of 1.4 if the temperature increases 10°C. This is sometimes called the Q10 for the process, and a Q10 of 1.4 is in line with a variety of observations from experiments. It is important to note once again that there is an upper limit to this temperature sensitivity function in the real world, but we do not expect to approach it in this modeling exercise, so we will not try to incorporate it into our equation.
Defined in the manner outlined above, our photosynthesis flow is the same as what is commonly called Gross Primary Production. Gross Primary Production differs from Net Primary Production, which is the gross minus respiration.
Plant Respiration
If we think of photosynthesis as the process of making fuel, then respiration can be thought of as the process of burning that fuel -- using it for maintenance and growth. This process can be described in the form of a reaction, just like photosynthesis. That reaction is:
CH2O + O2 Æ CO2 + H2O + energy
which is effectively the reverse reaction for photosynthesis. Through respiration, plants (and animals) release water and carbon dioxide. They also release energy, in the form of heat, or infrared radiation. Thus, the processes of living organisms represent a kind of energy flow system; energy flows in and energy of another form flows out. Does the energy involved in respiration and photosynthesis balance out as the equations seem to imply? The answer is no -- otherwise, how could organisms grow? This tells us that respiration does not equal photosynthesis for an individual plant or a whole community of plants. The difference goes into plant growth and operation.
Experiments on a variety of plants indicate that the ratio of photosynthesis to respiration is generally about 2 to 1, and this ratio does not appear to depend on the temperature. Or more precisely, the respiration does depend upon temperature, but in approximately the same way that photosynthesis does, so the ratio between them stays the same. When plants are young, and growing rapidly, but with not much biomass to maintain, this ratio is even higher; in older, larger plants, this ratio is lower since more carbon needs to go towards maintenance. For our purposes, we will assume that the global collection of plants has a ratio of around 2 to 1. More precisely, this ratio is 100 to 50; these are the current best estimates for the total amount of carbon, in gigatons, used and released in photosynthesis and respiration each year on a global basis.
For the purposes of our model, we will formulate plant respiration in the following manner:
Fpr = Fp * (50/100)
where Fpr is the rate of global plant respiration in Gt C/yr and Fp is the rate of global photosynthesis by land plants.
Litter Fall (and below-ground addition to the soil)
Dead plant material enters the soil in two ways -- it falls on the surface as litter, and it is contributed below the surface from roots. The relative importance of these two pathways into the soil carbon reservoir vary according to the plants in an ecosystem, but it appears that the two are commonly about equal, which may seem a bit surprising since loss of organic carbon from root systems is a process that we generally don't see. In our model, we will lump these processes together and call them litter fall, keeping in mind that this is only half of the real story (later, we can enhance our model by breaking the soil up into several different boxes, and these two flows will no longer be lumped together). Litter fall is set to be the difference between the photosynthetic uptake of carbon and the return of carbon through plant respiration. If this were not the case, then the size of the land biota reservoir would be growing or declining, which may in fact be the case (it's doing both in different parts of the Earth), but we would like our model to be more or less in a steady state to begin with. In reality, it is the litterfall that is actually measured in studies of carbon flow through ecosystems; that, combined with a measure of the gross primary productivity (the total amount of carbon used in photosynthesis) gives an estimate of the plant respiration flow according to the following equation:
Gross Primary Productivity = Plant Respiration + Litter Fall
Having already chosen the initial rates for photosynthesis and plant respiration, at 110 and 50, this leaves us with a value of 60 Gt C/yr for the rate of carbon added to the soil reservoir through the process of litter fall. But, we don't want this to be a constant value; it will undoubtedly change as a function of the size of the land biota reservoir. So, we can define this flow in the form of a standard draining flow:
Flf = land_biota * (50/INIT(land_biota)
where Flf is the flow in Gt C/yr and land_biota is the amount of carbon stored in the land biota (plants) at any given time.
Soil Respiration
Respiration also occurs within the soil, as microorganisms consume the dead plant material. In terms of a chemical formula, this process is the same as described above for plant respiration, only in this case, the microbes do not make the fuel themselves.
There is an unseen but fascinating universe of microbes living within the soil and they are the key means by which nutrients such as carbon and nitrogen are cycled through the soil system. A great diversity of microorganisms live in the soil -- perhaps as many as 1000 species in a cubic centimeter -- and they are capable of consuming tremendous quantities of organic material. Much of the organic material added to the litter (the accumulated material at the surface of the soil) or within the root zone each year is almost completely consumed by microbes; thus there is a reservoir of carbon with a very fast turnover time -- on the order of 1 to 3 years in many cases. The by-products of this microbial consumption are CO2, H2O, and a variety of other compounds, collectively known as humus. Humus is much less palatable compound, as far as microbes are concerned, and is not decomposed very quickly. After it is produced at shallow levels within the soil, it generally moves downward and accumulates in regions of the soil with high clay content. Part of the reason it accumulates in the lower parts of the soil is that there tends to be less oxygen in that environment, and the lack of oxygen makes it even more difficult for microbes to work on this humus and decompose it further. But eventually, due to various processes that stir the soil, this humus moves back up to where there is more oxygen and then the microbes will eventually destroy the humus and release some more CO2. This humus then constitutes another, longer-lived reservoir of carbon in the soil. 14C dates on some of this soil humus give ages of several hundred to a thousand years old. Taken together, the fast decomposition and the slower decomposition of humus, both driven by microbial processes, lead to an average residence time of around 20 to 30 years for most soils. The data used in our global carbon cycle model lead to a residence time of about 26 years for the soil carbon reservoir.
These microbes (considered in terms of their respiratory output) are very sensitive to the organic carbon content of the soil as well as the temperature and water content, respiring faster at higher carbon concentrations, higher temperatures and in moister conditions (although if the soil is flooded with water, conditions are worse since no oxygen can get into the soil -- the majority of the microbes need oxygen to respire, as can be seen from the general equation for respiration given under the discussion of plant respiration). Studies seem to indicate that the soil respiration is in fact even more sensitive to temperature than photosynthesis and plant respiration. In general, higher temperatures tend to correspond with higher rates of precipitation, so we can consider the affects of water to go hand in hand with temperature. In our model of the carbon cycle, we will use an expression for soil respiration that takes these observations into account:
The temperature sensitivity part of the equation is a linear function like that used in defining photosynthesis. The formulation here gives an increase in soil respiration by a factor of 2.0 if the temperature increases 10°C. This gives a Q10 of 2.0 is in line with a variety of observations. It is important to note once again that there is an upper limit to this temperature sensitivity function in the real world, but we do not expect to approach it in this modeling exercise, so we will not try to incorporate it into our equation.
Runoff
Although most of the carbon loss from the soil reservoir occurs through respiration, some carbon is transported away by water running off over the soil surface. This runoff is eventually transported to the oceans by rivers. The actual magnitude of this flow is a bit uncertain, although it does appear to be quite small. The most recent estimates place it at 0.6 Gt C/yr. In defining this flow in our model, we need to split it into the carbon added to the warm surface waters of the ocean and the carbon added to the cold surface waters. Since the warm waters of the ocean will be defined as covering 2/3 of the ocean surface, we will send 2/3 of 0.6, i.e., 4 Gt C/yr into the warm surface ocean and 1/3 into the cold surface ocean. The flows will furthermore be defined as standard draining processes (first-order kinetic processes) as follows:
where Frow and Froc are the runoff flows to the warm ocean and the cold ocean respectively.
Processes of Carbon Flow in the Oceanic Realm
Ocean - Atmosphere Exchange
Carbon dioxide can be dissolved in seawater, just as it can be dissolved in a can of soda; it can also be released from seawater as the CO2 from a soda can also be released. This transfer of gas back and forth between a liquid and the atmosphere is an extremely important process in the global carbon cycle since the oceans are such an enormous reservoir with the potential to store and release significant quantities of CO2. In general, this flow depends on the concentration of CO2 in the atmosphere and the oceans, but it gets a little complicated because the concentration of CO2 in seawater depends on a number of other factors. To understand this process, we need to have some sense of what happens to CO2 once it gets dissolved in seawater.
Carbonate Chemistry of Seawater
When CO2 enters seawater, it reacts with water and forms a series of products, as described in the following equation:
CO2 (gas) + H2O ´ H2CO3 ´ H+ + HCO3- ´ 2H+ + CO32-
This means that in seawater, you can find all of these different forms or species of carbon co-existing, but in reality, the bicarbonate (HCO3-) and carbonate (CO32-) ions are the most abundant, making up over 99% of the total dissolved carbon (see Figure 7.04.5).
In the above equation, the double-headed arrows mean that the
reactions can go in both directions and generally do until some
balance of the different compounds is achieved -- a chemical
equilibrium. Each of these reactions has associated with it an
equilibrium constant, which establishes the relative concentrations
of the compounds on either side of the reaction. For instance, if we
isolate two of the above reactions,
H2CO3 ´ H+ + HCO3- , and HCO3- ´ 2H+ + CO32-
we can say that the equilibrium constants, k1 and k2, are given by a ratio formed by the concentrations of the various compounds involved:
. (1)
We can do some algebra with these equations, just like normal equations. Our goal is to find an expression for the concentration of H2CO3, so we begin by rearranging the equation on the left above (1) to the following form:
. (2)
Then we rearrange the right side of (1) so that it becomes:
. (3)
Next, we substitute (3) into (2), to give us our desired equation expressing H2CO3 in terms of HCO3- and CO32-.
. (4)
Ultimately, we want to get an expression for the concentration of CO2 gas contained in the seawater at equilibrium; this is usually expressed as the partial pressure of CO2, with units of matm (microatmospheres), or ppm (parts per million, by volume) rather than a typical concentration, which would have units of moles per cubic meter of water. The partial pressure is given by the following formula:
, (5)
in which K3 is a slightly different kind of equilibrium constant that incorporates what is called the solubility of CO2 in seawater, which is a function of temperature and salinity. Our next step is to combine the various equilibrium constants into a single value as:
(6)
keeping in mind that this value will be a function of temperature (also salinity). Then we can substitute (4) and (6) into (5) to obtain another expression for the partial pressure of carbon dioxide gas in seawater:
. (7)
The next thing we need to do is to find expressions for the concentrations of carbonate and bicarbonate in terms of the total amount of carbon dissolved in seawater, which will change over time as the oceans release or absorb CO2 from the atmosphere. First, we need to define a term for the total concentration of inorganic carbon in solution:
. (8)
This is an approximation since it ignores CO2 gas and H2CO3, but both of these are very minor components. This sum, the concentration of total dissolved carbon, is simply equal to the amount of carbon in the ocean reservoir divided by the volume of the ocean. Looking at the above equation, we see that it does not tell us the relative proportions of the carbonate and bicarbonate -- just their combined concentration. So, we need to find some other means of establishing the proportions of these two forms of carbon.
We get some help from the fact that the relative proportions of bicarbonate (HCO3-) and carbonate (CO32-) play an important role in establishing the balance of positive and negative charges in seawater. Ions like calcium (Ca2+), sodium (Na+), , potassium (K+), and magnesium (Mg2+) all add positive charges to seawater; these are partially countered by chloride (Cl-), sulfate (SO42-), nitrate (NO3-), and bromide (Br-) ions, but the result is a slight deficit of negatively charged ions. This difference is essentially made up by the carbonate and bicarbonate ions. If more negative charge is needed, then more of the carbon occurs in the form of carbonate since it has a charge of minus 2, while if less negative charge is needed, more of the carbon exists in the form of bicarbonate.
The excess positive charge of seawater that needs to be balanced by the different forms of dissolved carbon is called the alkalinity of the seawater. The alkalinity is defined as:
. (9)
Note that the carbonate ion counts twice since it has a minus 2 charge. It is important to realize that the total alkalinity is really determined by the other ions in solution -- the ones mentioned above. This means that for the purposes of our model, we can keep the alkalinity constant, although we will eventually define slightly different alkalinities for the warm and cold surface waters of the oceans. Looking at the above equation, you can see that with a given alkalinity, if we have just a little dissolved carbon, more of the carbon will be in the form of the carbonate ion (CO32-) in order to make up the charge imbalance, but if we have a high concentration of dissolved carbon, there will be a greater proportion of the bicarbonate ion (HCO3-). The concentrations of both the carbonate and bicarbonate ions can be expressed as a function of both the alkalinity and the concentration of total dissolved inorganic carbon, SCO2, as shown in the following:
. (10)
Here, we need to remember that this is an approximation because we ignored the term for H2CO3 in equation (8). In the model we will eventually experiment with, we use a more precise formulation that does not ignore the concentration of H2CO3. This increases the complexity of the algebra, giving us a quadratic equation whose solution ends up as:
. (11)
Regardless of whether we consider the more digestible form (10) or the more precise form of expressing HCO3- and CO32- (11), we are finally set, because if we look at the equation for the partial pressure of CO2,
we see that all of the terms can be expressed in terms of components of the model. We have reached the light at the end of the algebraic tunnel.
As can be seen, the chemistry of carbon in seawater is relatively complex, but it turns out to be extremely important in governing the way the global carbon cycle operates. Figure 7.05 illustrates some of these complexities in the form of a series of graphs made by the model in which we have varied just one parameter at a time and then monitored its effect on the pCO2 of seawater.
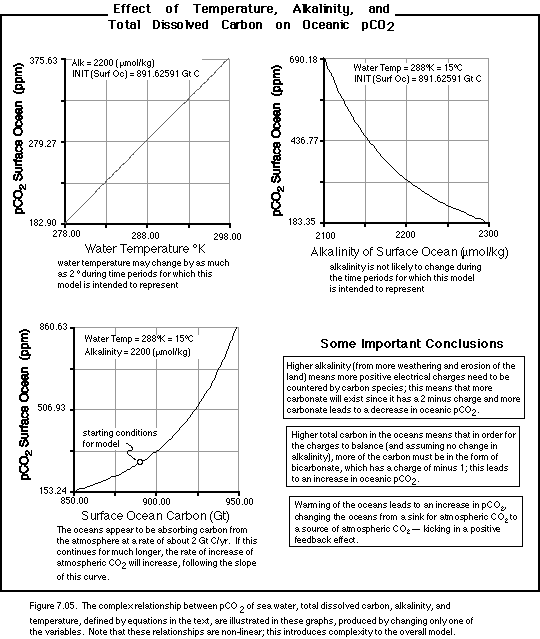
Summary of Carbonate Chemistry
Let's see if we can summarize this carbonate chemistry -- it is important to have a good grasp of this if we are to understand how the global carbon cycle works.
• Carbon can exist in three main inorganic forms in sea water -- CO2, HCO3-, and CO32-, and there is a rapidly-achieved equilibrium between these species.• The ratio of HCO3- to CO32- along with the water temperature determines the CO2 concentration of seawater. • The alkalinity of sea water represents the positively-charged ions that need to be countered by negatively-charged carbonate ions.
• The concentration of the total dissolved inorganic carbon, along with the alkalinity determines the ratio of HCO3- to CO32-, and thus the CO2 concentration of seawater.
• The CO2 concentration of seawater, relative to the atmospheric CO2, determines whether the oceans can absorb or release CO2 .
• The ability of carbon to switch back and forth between these three forms means that only a portion of the CO2 absorbed by the oceans will remain as CO2.
We now move on to find an expression for the actual rate of transfer of CO2 back and forth across the interface between the air and the oceans.
Ocean - Atmosphere Exchange Revisited
The exchange of gas between the air and the sea is, in theory, governed by the rate of diffusion and the distance the diffusion has to cover, as indicated in figure 7.06.
As you can imagine, it is pretty hard to measure the thickness of the stagnant film, so instead, geochemists have taken another approach to this problem -- find a coefficient that accurately describes the observed rate of gas transfer between the oceans and the atmosphere. This coefficient, which we will consider to be a constant, is:
How has this coefficient been determined? Several methods have been used, but one of the more interesting involves the use of 14C that was generated by atmospheric nuclear explosion tests. These tests stopped in about 1963, and since that time, the abundance of 14C in the atmosphere has steadily declined. Part of the decline is due to radioactive decay, but a major part of the decline is due to absorption by the oceans. The abundance of 14C in seawater, and its distribution with depth are observations that provide enough information to determine the coefficient of gas transfer. This effectively amounts to using the bomb-generated 14C as a tracer in the sea -- an unexpected benefit from the nuclear weapons program.
This coefficient then allows us to finally get a simple expression for the rate of gas transfer that we can use in our model of the global carbon cycle:
Fatm-oc = koa * (pCO2atm - pCO2oc) * Aoc
where Aoc is the surface area of the ocean and the pCO2 values for the atmosphere and ocean are expressed in units of ppm. The flow units are grams of carbon per year, which can then be converted to Gigatons of carbon per year, using the relationship that 1Gt = 1 x 1015g.
In our more complex model, the surface of the ocean is broken up into two regions that are treated separately, so we will have two equations for the gas exchange between the surface ocean and the atmosphere. In doing this, we try to represent the observation that the warm parts of the oceans give up CO2 to the atmosphere while the colder parts absorb CO2 from the atmosphere.
Figure 7.07, shows the difference between atmospheric and ocean surface CO2 concentrations; positive numbers represent warm areas where the oceans give up CO2 and negative values represent areas where the oceans absorb CO2.

Advection -- Surface Currents
The wind-driven circulation of the surface waters of the ocean means that there is a mixing or exchange of water between the warm and cold regions of the ocean surface. This process is here referred to as advection -- similar to the advection that we included in the model of the global water cycle. In the oceans, warm surface waters move up to the polar regions and are added to the cold surface waters there, while some of the cold surface water returns back to the equatorial regions, making a large-scale cycle that is important for the transfer of heat energy, as we discussed in our earlier work with energy balance climate models. It turns out that in terms of net carbon transport, the flow from the warm part of the ocean to the colder part is greater than the return, so we will model this process as a unidirectional flow from warm to cold. But how can this be the case? Wouldn't the amount of carbon in the cold surface waters increase substantially in a short period of time? The answer is, yes, it would, except for the fact that there are other flows of carbon out of the this cold water reservoir, most importantly, downwelling. Downwelling will be discussed in more detail below, but it does indeed balance this addition from advection.
The actual amount of carbon transported through advection is not very well constrained, but here we assign it a value of 10 Gt C/yr, which is in the range of some other estimates. This flow will likely vary according to changes in the circulation pattern of the worlds oceans, and also as a function of the concentration of dissolved carbon in the seawater. In other words, the circulation controls how many cubic meters of water are transferred from the warm to the cold region, and the concentration of carbon controls how many grams or moles of carbon are contained within each cubic meter. For the sake of simplicity, and since we don't really have any better information to go on, we will represent this flow as a fixed percentage of the amount of carbon contained in the warm surface water reservoir. This makes this flow a first-order kinetic process, like many of the other flows in this model. In the form of an equation:
where Fadv is the flow of carbon by advection and WarmSurfOc is the reservoir of carbon stored in the warm surface waters of the worlds oceans.
Marine Biota Exchange -- The Biologic Pump
The surface waters of the worlds oceans are home to a great number of organisms that have as the basis for their food web photosynthesizing phytoplankton. These plants utilize CO2 gas dissolved in seawater and turn it into organic matter, and just like land plants, these phytoplankton also respire, returning CO2 to the surface waters. At the same time, many planktonic organisms extract dissolved carbonate ions from seawater and turn them into CaCO3 shells. When these planktonic organisms die, their soft parts are mainly consumed and decomposed very quickly, before they can settle out into the deeper waters of the oceans. This decomposition thus returns carbon, in the form of CO2, to seawater. However, some of the organic remains and the inorganic calcium carbonate shells will sink down into the deep oceans, thus transferring carbon from the shallow surface waters into the huge reservoir of the deep oceans. This transfer is often referred to as the biologic pump, and it causes the concentration of CO2 gas, and also SCO2 , the concentration of total dissolved inorganic carbon in the surface waters to be less than that of the deeper waters. This can be seen in Figure 7.08, which shows the vertical distribution of SCO2 (also alkalinity) in a profile view for some of the major regions of the world's oceans.
Why is the alkalinity reduced in the surface waters? For the same
reason that SCO2
is depleted. Planktonic organisms make shells of
CaCO3,
and when these sink to the seafloor, they carry Ca2+
ions with them, thus reducing the alkalinity. Much of this
CaCO3
is later dissolved when it reaches deeper parts of the oceans, which
explains the higher alkalinity values in the deep waters, as seen in
Figure 7.08. By controlling the concentration of CO2 gas
dissolved in the surface waters, the planktonic organisms exert a
strong influence on the concentration of CO2 in the
atmosphere. For instance, if the biologic pump were turned off,
atmospheric CO2 would rise to about 500 ppm (relative to
the current 350 ppm); if the pump were operating at maximum strength
(i.e., complete utilization of nutrients), atmospheric CO2
would drop to a low of 140 ppm. Clearly, this biologic pump is an
important process.
What controls the strength of this biologic pump? The photosynthesizing plankton require nutrients in addition to CO2 in order to thrive; specifically, they require nitrogen and phosphorus. Most of these plants need P, N, and C in a ratio of 1:16:125 and since at present, the ratio of P to N in ocean water is about 1:16, both P and N limit the growth of these phytoplankton. Photosynthetic activity of plankton can be mapped out by satellites tuned to record differences in water color due to the presence of chlorophyll. This distribution is shown in Figure 7.09 below.
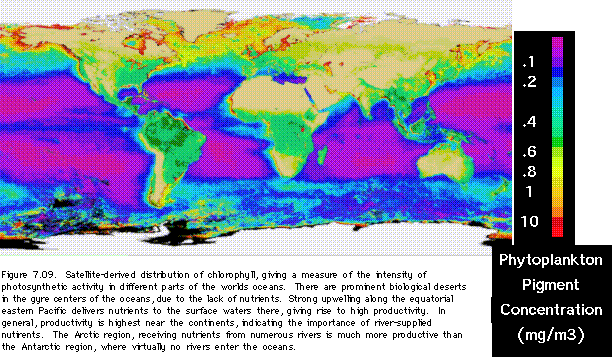
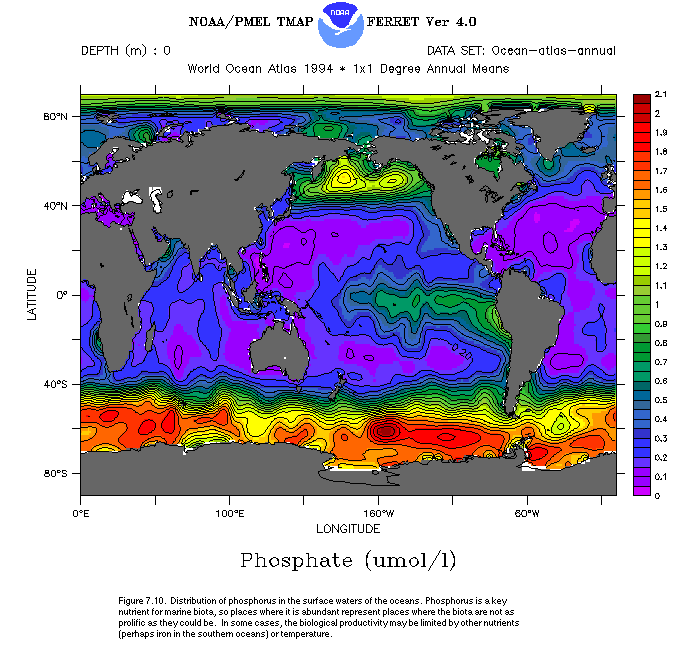
The most straight-forward way to represent the function of the marine biota in our model would be to represent the marine biota in the form of two reservoirs, one for each of the two surface water reservoirs. The flow of carbon in and out of these marine biota reservoirs would be represented by three flows -- a photosynthetic uptake flow, a respiration flow (which combines plant respiration with rapid decomposition within the upper 100 m of water), and a sinking flow. The photosynthetic uptake flow is really controlled by the availability of nutrients and the water temperature, but since we are not keeping track of nutrients or the temperature of the water, we will take a far simpler approach.
The primary reason for developing a simpler approach is that the sizes of the marine biota reservoirs are so small relative to the flows in and out -- this would force us to have a very small time step (about 0.05 yrs) for our model simulations, and we would have a very slow model as a result. The way around this is to simply ignore the reservoirs that represent the marine biota, and replace the three flows for each marine biota reservoir with one single flow that transfers carbon from the surface water reservoirs to the deep water reservoirs. We will call these flows the warm and cold biologic pumps and to begin with, they will simply be represented by constant flows of 6 Gt C/yr from the warm water, and 4 Gt C/yr from the cold surface water. Adopting this more stream-lined approach also avoids the problem of keeping track of limiting nutrients like P and N.
Downwelling
As mentioned above, downwelling transfers cold surface waters into the deep interior of the oceans, and as a result, carbon is transferred as well. The magnitude of the flow is thus a function of the volume of water flowing and the average concentration of carbon in the cold surface waters, which is itself a function of the total amount of carbon stored in this reservoir, assuming that the size of the reservoir is not changing appreciably over the few hundred years that this model is intended to be used for.
Downwelling occurs primarily near the poles, where surface waters are strongly cooled by contact with the air. This cooling leads to a density increase. The formation of ice from seawater at the margins of Antarctica increases the salinity of the seawater there, adding to the density of the water. This dense water then sinks and flows through the deep oceans, effectively mixing them on a timescale of about 1000 years or so (the Atlantic Ocean mixes somewhat faster, which helps explain the smaller SCO2 and alkalinity gradients seen in Figure 7.08).
In our model, the flow of carbon from the cold, shallow water to deep water will simply be a function of how much carbon is stored in the cold surface water reservoir. This implies that we are not altering the volumetric flow of downwelling water, which is probably acceptable if we are only concerned with a few hundred years. In the form of an equation, this flow is:
where Fdw is the flow of carbon due to downwelling, in Gt C/yr. This equation is set so that at the beginning of the model, the magnitude of this flow will be 96.2, which is within the range of current estimates, adjusted here to make the system be in a steady state.
Upwelling
Upwelling is the converse of downwelling, and as the deep waters rise to the surface, they bring with them carbon. The total transfer of carbon is thus a function of the volume of water involved in this flow and the amount of carbon stored in the deep ocean reservoir. Upwelling occurs in areas of the oceans where winds and surface currents diverge, moving the surface waters away from a region; in response, deep waters rise up to fill the "void". Upwelling occurs along the equator, where there is a strong divergence, and also along the margins of some continents, such as the west coast of South America. This upwelling water also brings with it nutrients such as nitrogen and phosphorus, making these waters highly productive.
We will define the flow of carbon resulting from upwelling in a like manner to the downwelling flow:
Note that the amount of carbon transferred by this flow is greater than the downwelling flow. This is not because the volume of flow is different in these two processes, but rather because the concentration of carbon in the deep waters of the ocean is greater than that in the shallow surface waters, due in part to the operation of the biologic pump mentioned above.
Sedimentation
Some of the carbon, both organic and inorganic (i.e., calcium carbonate shells) produced by marine biota and transferred to the deep oceans settles out onto the sea floor and accumulates there, eventually forming sedimentary rocks. The magnitude of this flow is small -- about 0.6 Gt C/yr -- relative to the total amount transferred by sinking from the surface waters -- 10 Gt C/yr. The reason for this difference is primarily because the deep waters of the oceans dissolve calcium carbonate shell materials; below about 4 km, the water is so corrosive that virtually no calcium carbonate material can accumulate on the sea floor. In addition, some of the organic carbon is consumed by organisms living in the deep waters and within the sedimentary material lining the sea floor. This consumption results in the release of CO2 into the bottom waters and thus decreases the amount of carbon that is removed from the ocean through this process. It is worth noting that the process of organic carbon consumption on the seafloor is another microbial process and is very similar to the soil respiration flow described above. Since the microbes living on the seafloor require oxygen to accomplish this task, the supply of oxygen to the seafloor by deep currents is an important part of this process.
We will define the sedimentation flow to be a fixed percentage of the amount of carbon transferred to the deep waters by the biologic pump flows from the shallow marine biota reservoirs. The equation for this flow is thus:
,
where Fsed is the transfer of carbon from the deep ocean reservoir to the sedimentary rock reservoir, and Fswob and Fscob are the flows of carbon from the warm and cold surface ocean biota. This equation is set up so that initially, with Fswob and Fscob set to total 10 Gt C/yr, the Fsed flow will have a value of 0.6 Gt C/yr.
Volcanism and Metamorphism
When sedimentary rocks deposited on oceanic crust are subducted, they may melt or undergo metamorphism; in either case, the carbon stored in calcium carbonate -- limestone -- is liberated in the form of CO2, which ultimately is released at the surface. The CO2 may come out when a volcano erupts, or it may slowly diffuse out from the interior via hot springs, but in both cases, it represents a transfer of carbon from the reservoir of sedimentary rocks to the atmosphere. The magnitude of this flow is quite small, and is adjusted here to a value of 0.6 Gt C/yr in order to create a model in steady state. This flow is defined as a constant in the model, although in reality, it will vary according to the timing of large volcanic eruptions. An extremely large volcanic eruption may emit carbon at a rate of around 0.2 Gt C/yr for a year or two, creating a minor fluctuation.
Processes of Carbon Flow in the Human Realm
Fossil Fuel Burning
Another pathway for carbon to move from the sedimentary rock reservoir to the atmosphere is through the burning of fossil fuels by humans. Fossil fuels include petroleum, natural gas, and coal, all of which are produced by slow transformation of organic carbon deposited in sedimentary rocks -- essentially the fossilized remains of marine and land plants. In general, this transformation takes many millions of years; most of the oil and gas we now extract from sedimentary rocks is on the order of 70-100 million years old. These fossil fuels are primarily composed of carbon and hydrogen. For instance, methane, the main component of natural gas, has a chemical formula of CH4; petroleum can be represented by the formula of CH2. The combustion of fossils fuels involves the use of oxygen and the release of carbon dioxide and water, as represented by the following description of burning petroleum:
2CH2 + 3O2 Æ 2CO2 + 2H2O.
Beginning with the onset of the industrial revolution at the end of the last century, humans have been burning increasing quantities of fossil fuels as our primary energy source. As a consequence, the amount of CO2 emitted from this burning has undergone an exponential rise that follows the exponential rise in the human population. The magnitude of this flow is currently about 5 to 6 Gt C/yr. This number also includes the CO2 generated in the production of cement, where limestone is burned, liberating CO2.
To begin with, this flow will be ignored in our model, and we will add it in later, after we have a model that is in a steady state so that we can better assess the impact of fossil fuel burning.
Land-Use Changes -- Forest Burning and Soil Disruption
The other form of human alteration of the global carbon cycle is through forest cutting and burning and the disruption of soils. When deforestation occurs, most of the plant matter is either left to decompose on the ground or it is burned, the latter being the more common occurrence. This process reduces the size (the mass) of the land biota reservoir and the burning adds carbon to the atmosphere. Land-use changes other than deforestation can also add carbon to the atmosphere; agriculture, for instance, involves tilling the soil, which leads to very rapid decomposition and oxidation of soil organic matter. This means that in terms of a system, we are talking about two separate flows here -- one draining the land biota reservoir, the other draining the soil reservoir; both flows transfer carbon to the atmosphere. Current estimates place the total addition to the atmosphere from forest burning and soil disruption at around 1.5 Gt C/yr; estimates divide this into 70% to 50% forest burning, with soil disruption making up the remainder.
As with fossil fuel burning, the forest burning and soil disruption flows will not be included in our initial model; we will add them to a steady-state model in order to figure out how these processes affect the global carbon cycle.
Readings and Key References:
- Broecker, W.S., and Peng, H.-S., 1993, Greenhouse Puzzles, New York, Eldigio Press, 251 p.
- Gifford, R.M., 1993, Implications of CO2 effects on vegetation for the global carbon budget, in, Heimann, M., (ed.), The Global Carbon Cycle, NATO ASI Series, v. 115, p. 159-200.
- Holmen, K., 1992, The Global Carbon Cycle, in, Butcher, S., Charlson, R., Orians, G., and Wolfe, G., (eds.), Global Biogeochemical Cycles, London, Academic Press, p. 237-262.
- IPCC, 1995, Climate Change 1994, Cambridge, Cambridge University Press, 339 p., but mainly p. 35-71.
- Kump, L., 1991, Interpreting carbon-isotope excursions: Strangelove oceans, Geology, v. 299, 302.
- Kwon, O.-Y., and Schnoor, J.L., 1994, Simple global carbon model: the atmosphere-terrestrial biosphere - ocean interaction, Global Biogeochemical Cycles, v. 8, p. 295-305.
- Post, W.M., Peng, H.-S., Emanuel, W.R., King, A.W., Dale, V.H., and DeAngelis, D. L., 1990, The global carbon cycle, American Scientist, v. 78, p. 310-326.
- Siegenthaler, U., and Sarmiento, J.L., 1993, Atmospheric carbon dioxide and the ocean, Nature, v. 365, p. 119-125.
- Walker, J.C.G., 1991, Numerical Adventures with Geochemical Cycles, Oxford, Oxford University Press, 192 p.